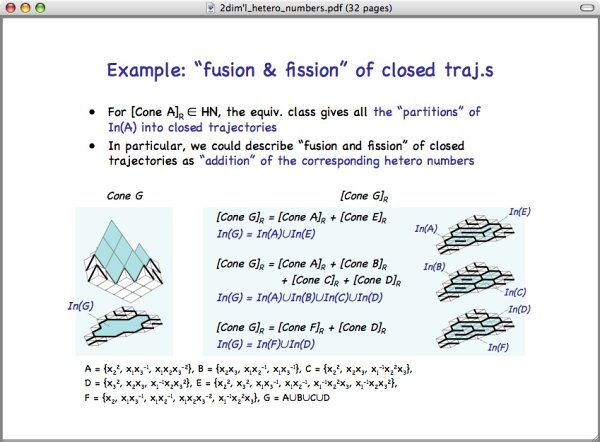
Finally, as an example of hetero numbers, let’s consider “fusion and fission” of closed trajectories.
For hetero number [Cone A]R, the cones in the equivalent class gives all the …
In particular, we could describe …, as shown below.
Shown on the left is Cone G and its associated conjugate roof (colored blue). They specify the shape of In(G) on the hyperplane. The contents of G and others are given below.
Shown on the right is all the cones in the equivalent class of Cone G. As you see, there are three cones:
The first one is associated with two closed trajectories which have origin in Cone A and Cone E respectively. Thus, it corresponds to [Cone A]R + [Cone E]R. And the partition of In(G) into two closed trajectories, In(A) and in(E), is expressed as addition
[Cone G]R = [Cone A]R + [Cone E]R.
The second one is associated with four closed trajectories which have origin in Cone A , Cone B, Cone C,and Cone D respectively. Thus, it corresponds to [Cone A]R + [Cone B]R + [Cone C]R + [Cone D]R. And the partition of In(G) into four closed trajectories, In(A), In(B), In(C), and in(D), is expressed as addition
[Cone G]R = [Cone A]R + [Cone B]R + [Cone C]R + [Cone D]R.
And the last one is associated with two closed trajectories which have origin in Cone F and Cone D respectively. Thus, it corresponds to [Cone F]R + [Cone D]R. And the partition of In(G) into two closed trajectories, In(F) and in(D), is expressed as addition
[Cone G]R = [Cone F]R + [Cone D]R.